Let's Do Maths! Mean, Median, Mode & Range.
- ptessslmc

- Oct 15, 2020
- 3 min read

Mean, median, and mode
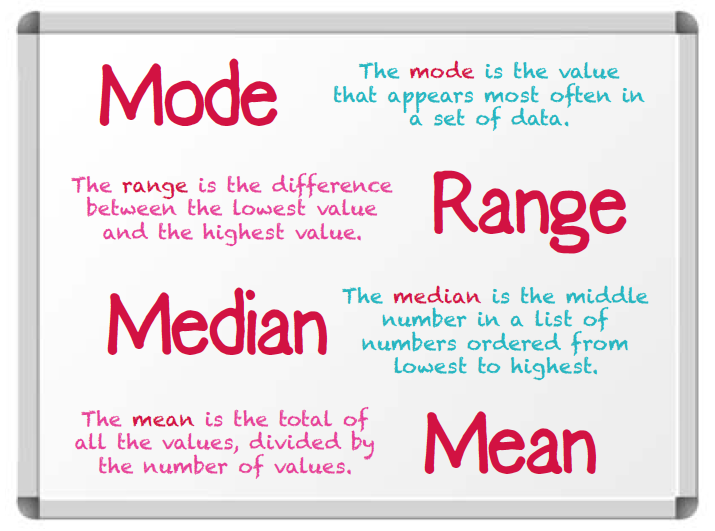
Mean, median, and mode are different measures of center in a numerical data set. They each try to summarize a dataset with a single number to represent a "typical" data point from the dataset.
Mean: The "average" number; found by adding all data points and dividing by the number of data points.
Example: The mean of 444, 111, and 777 is (4+1+7)/3 = 12/3 = 4(4+1+7)/3=12/3=4left parenthesis, 4, plus, 1, plus, 7, right parenthesis, slash, 3, equals, 12, slash, 3, equals, 4.
Median: The middle number; found by ordering all data points and picking out the one in the middle (or if there are two middle numbers, taking the mean of those two numbers).
Example: The median of 444, 111, and 777 is 444 because when the numbers are put in order (1(1left parenthesis, 1, 444, 7)7)7, right parenthesis, the number 444 is in the middle.
Mode: The most frequent number—that is, the number that occurs the highest number of times.
Example: The mode of \{4{4left brace, 4, 222, 444, 333, 222, 2\}2}2, right brace is 222 because it occurs three times, which is more than any other number.

Calculating the Mean
There are many different types of mean, but usually when people say mean, they are talking about the arithmetic mean.
The arithmetic mean is the sum of all of the data points divided by the number of data points.
mean=sum of data# of data points
Here's the same formula written more formally:
\text{mean}=\dfrac{\sum{x_i}}{n}mean=n∑xistart text, m, e, a, n, end text, equals, start fraction, sum, x, start subscript, i, end subscript, divided by, n, end fraction
Example
Find the mean of this data: 111, 222, 444, 555
Start by adding the data: 1+2+4+5=121+2+4+5=121, plus, 2, plus, 4, plus, 5, equals, 12
There are 444 data points.
\text{mean}=\dfrac{12}{4}=3mean=412=3start text, m, e, a, n, end text, equals, start fraction, 12, divided by, 4, end fraction, equals, 3
The mean is 333.
Finding the median
The median is the middle point in a dataset—half of the data points are smaller than the median and half of the data points are larger.
To find the median:
Arrange the data points from smallest to largest.
If the number of data points is odd, the median is the middle data point in the list.
If the number of data points is even, the median is the average of the two middle data points in the list.
Example 1
Find the median of this data: 111, 444, 222, 555, 000
Put the data in order first: 000, 111, 222, 444, 555
There is an odd number of data points, so the median is the middle data point.
000, 111, \large222, 444, 555
The median is 222.
Example 2
Find the median of this data: 101010, 404040, 202020, 505050
Put the data in order first: 101010, 202020, 404040, 505050
There is an even number of data points, so the median is the average of the middle two data points.
101010, \large{20}2020, \large{40}4040, 505050
\text{median} = \dfrac{20+40}{2}=\dfrac{60}{2}=30median=220+40=260=30start text, m, e, d, i, a, n, end text, equals, start fraction, 20, plus, 40, divided by, 2, end fraction, equals, start fraction, 60, divided by, 2, end fraction, equals, 30
The median is 303030.
Finding the mode
The mode is the most commonly occurring data point in a dataset. The mode is useful when there are a lot of repeated values in a dataset. There can be no mode, one mode, or multiple modes in a dataset.
Example 1
Ms. Norris asked students in her class how many siblings they each had.
Find the mode of the data: 000, 000, 111, 111, 111, 111, 111, 111, 222, 222, 222, 333, 555
Look for the value that occurs the most: 000, 000, \large111, \large111, \large111, \large111, \large111, \large111, 222, 222, 222, 333, 555
The mode is 111 sibling.
Example 2
Ms. Rubin asked students in her class how many siblings they each had.
Find the mode of the data: 000, 000, 000, 111, 111, 111, 111, 222, 222, 222, 222, 444
Look for the value that occurs the most: 000, 000, 000, \large111, \large111, \large111, \large111, \large222, \large222, \large222, \large222, 444
There is a tie for the value that occurs the most often.
The modes are 111 and 222 siblings.
Finding the Range



Comments